COLLIGATIVE PROPERTIES OF SOLUTION

Introduction and Summary

Solutions, especially liquid solutions, generally have markedly different properties than either the pure solvent or the solute. For example, a solution of sugar in water is neither crystalline like sugar nor tasteless like water. Some of the properties unique to solutions depend only on the number of dissolved particles and not their identity. Such properties are called colligative properties. The colligative properties we will consider in this SparkNote are vapor pressure lowering, freezing point depression, boiling point elevation, and osmotic pressure.

When a nonvolatile solute is dissolved in a solvent, the vapor pressure of the resulting solution is lower than that of the pure solvent. The amount of the vapor pressure lowering is proportional to the amount of solute and not its identity. Therefore, vapor pressure lowering is a colligative property. The equation that describes that phenomenon is called Raoult's law.

Boiling point elevation is a colligative property related to vapor pressure lowering. The boiling point is defined as the temperature at which the vapor pressure of a liquid equals the atmospheric pressure. Due to vapor pressure lowering, a solution will require a higher temperature to reach its boiling point than the pure solvent.

Every liquid has a freezing point--the temperature at which a liquid undergoes a phase change from liquid to solid. When solutes are added to a liquid, forming a solution, the solute molecules disrupt the formation of crystals of the solvent. That disruption in the freezing process results in a depression of the freezing point for the solution relative to the pure solvent.

When a solution is separated from a volume of pure solvent by a semi-permeable membrane that allows only the passage of solvent molecules, the height of the solution begins to rise. The value of the height difference between the two compartments reflects a property called the osmotic pressure of a solution. As you know, if you add more solvent to a solution, the two mix together to form a more dilute solution. The same forces allowing that mixing serve to force solvent molecules from the pure solvent compartment across the membrane into the solution compartment causing the change in volume. The amount of osmotic pressure is directly related to the concentration of the solute. That is because more concentrated the solutions have greater potentials for dilution.

Terms

Colligative Property - A property that depends only on the amount of solute in a solution and not the identity of the solute.

Concentrated - A solution of high concentration.

Concentration - The amount of solute dissolved in a given amount of solvent.

Dilute - A solution of low concentration or the process of lowering the concentration of a solution by the addition of solvent.

Dilution - The decrease of the concentration of a solution by the addition of more solvent.

Dissolve - To become a part of a solution.

Nonvolatile - A substance that does not have an appreciable vapor pressure.

Raoult's Law - The vapor pressure of a solution is the product of the mole fraction of the solvent and the vapor pressure of the pure solvent.

Solubility - The amount of a particular solute that can dissolve in a given amount of a particular solvent. Solubilities are generally listed in g / L.

Solute - A minor component of a solution.

Solution - A homogeneous mixture.

Solvent - The major component of a solution.

Colligative Properties

What are Colligative Properties?

A we have discussed, solutions have different properties than either the solutes or the solvent used to make the solution. Those properties can be divided into two main groups--colligative and non-colligative properties. Colligative properties depend only on the number of dissolved particles in solution and not on their identity. Non-colligative properties depend on the identity of the dissolved species and the solvent.

To explain the difference between the two sets of solution properties, we will compare the properties of a 1.0 M aqueous sugar solution to a 0.5 Msolution of table salt (NaCl) in water. Despite the concentration of sodium chloride being half of the sucrose concentration, both solutions have precisely the same number of dissolved particles because each sodium chloride unit creates two particles upon dissolution--a sodium ion, Na⁺, and a chloride ion, Cl^-. Therefore, any difference in the properties of those two solutions is due to a non-colligative property. Both solutions have the same freezing point, boiling point, vapor pressure, and osmotic pressure because those colligative properties of a solution only depend on the number of dissolved particles. The taste of the two solutions, however, is markedly different. The sugar solution is sweet and the salt solution tastes salty. Therefore, the taste of the solution is not a colligative property. Another non-colligative property is the color of a solution. A 0.5 M solution of CuSO₄ is bright blue in contrast to the colorless salt and sugar solutions. Other non-colligative properties include viscosity, surface tension, and solubility.

Raoult's Law and Vapor Pressure Lowering

When a nonvolatile solute is added to a liquid to form a solution, the vapor pressure above that solution decreases. To understand why that might occur, let's analyze the vaporization process of the pure solvent then do the same for a solution. Liquid molecules at the surface of a liquid can escape to the gas phase when they have a sufficient amount of energy to break free of the liquid's intermolecular forces. That vaporization process is reversible. Gaseous molecules coming into contact with the surface of a liquid can be trapped by intermolecular forces in the liquid. Eventually the rate of escape will equal the rate of capture to establish a constant, equilibrium vapor pressure above the pure liquid.

If we add a nonvolatile solute to that liquid, the amount of surface area available for the escaping solvent molecules is reduced because some of that area is occupied by solute particles. Therefore, the solvent molecules will have a lower probability to escape the solution than the pure solvent. That fact is reflected in the lower vapor pressure for a solution relative to the pure solvent. That statement is only true if the solvent is nonvolatile. If the solute has its own vapor pressure, then the vapor pressure of the solution may be greater than the vapor pressure of the solvent.

Note that we did not need to identify the nature of the solvent or the solute (except for its lack of volatility) to derive that the vapor pressure should be lower for a solution relative to the pure solvent. That is what makes vapor pressure lowering a colligative property--it only depends on the number of dissolved solute particles.

summarizes our discussion so far. On the surface of the pure solvent (shown on the left) there are more solvent molecules at the surface than in the right-hand solution flask. Therefore, it is more likely that solvent molecules escape into the gas phase on the left than on the right. Therefore, the solution should have a lower vapor pressure than the pure solvent.

Figure %: The Vapor Pressure of a Solution is Lower than that of the Pure Solvent

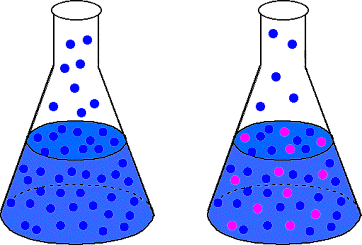
The French chemist Francois Raoult discovered the law that mathematically describes the vapor pressure lowering phenomenon. Raoult's law is given in :

Figure %: Raoult's Law Describes the Mathematics of Vapor Pressure Lowering

Raoult's law states that the vapor pressure of a solution, P, equals the mole fraction of the solvent, c _solvent, multiplied by the vapor pressure of the pure solvent, P^o. While that "law" is approximately obeyed by most solutions, some show deviations from the expected behavior. Deviations from Raoult's law can either be positive or negative. A positive deviation means that there is a higher than expected vapor pressure above the solution. A negative deviation, conversely, means that we find a lower than expected vapor pressure for the solution. The reason for the deviation stems from a flaw in our consideration of the vapor pressure lowering event--we assumed that the solute did not interact with the solvent at all. That, of course, is not true most of the time. If the solute is strongly held by the solvent, then the solution will show a negative deviation from Raoult's law because the solvent will find it more difficult to escape from solution. If the solute and solvent are not as tightly bound to each other as they are to themselves, then the solution will show a positive deviation from Raoult's law because the solvent molecules will find it easier to escape from solution into the gas phase.

Solutions that obey Raoult's law are called ideal solutions because they behave exactly as we would predict. Solutions that show a deviation from Raoult's law are called non-ideal solutions because they deviate from the expected behavior. Very few solutions actually approach ideality, but Raoult's law for the ideal solution is a good enough approximation for the non- ideal solutions that we will continue to use Raoult's law. Raoult's law is the starting point for most of our discussions about the rest of the colligative properties, as we shall see in the following section.

Boiling Point Elevation

One consequence of Raoult's law is that the boiling point of a solution made of a liquid solvent with a nonvolatile solute is greater than the boiling point of the pure solvent. The boiling point of a liquid or is defined as the temperature at which the vapor pressure of that liquid equals the atmospheric pressure. For a solution, the vapor pressure of the solvent is lower at any given temperature. Therefore, a higher temperature is required to boil the solution than the pure solvent. is a phase diagram for both a pure solvent and a solution of that solvent and a nonvolatile solute that explains that point graphically.

Figure %: Phase Diagram for a Solvent and its Solution with a Nonvolatile Solute

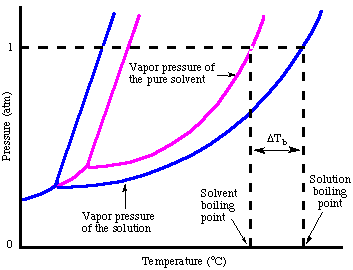
As you can see in the the vapor pressure of the solution is lower than that of the pure solvent. Because both pure solvent and solution need to reach the same pressure to boil, the solution requires a higher temperature to boil. If we represent the difference in boiling point between the pure solvent and a solution as ΔT_b, we can calculate that change in boiling point from the :

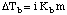
In the we use the units molality, m, for the concentration, m, because molality is temperature independent. The term K_b is a boiling point elevation constant that depends on the particular solvent being used. The term i in the above equation is called the van't Hoff factor and represents the number of dissociated moles of particles per mole of solute. The van't Hoff factor is 1 for all non-electrolyte solutes and equals the total number of ions released for electrolytes. Therefore, the value of i for Na₂SO₄ is 3 because that salt releases three moles of ions per mole of the salt.

Freezing Point Depression

As you may have noticed when we looked at the , the freezing point is depressed due to the vapor pressure lowering phenomenon. The points out that fact:

Figure %: Phase Diagram for a Solution and the Pure Solvent Indicating the Freezing Point Depression

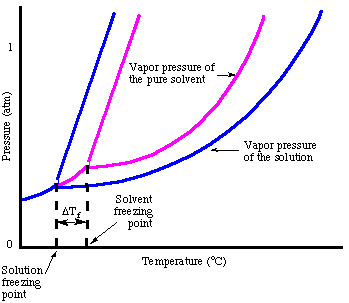
In analogy to the boiling point elevation, we can calculate the amount of the freezing point depression with the :

Note that the sign of the change in freezing point is negative because the freezing point of the solution is less than that of the pure solvent. Just as we did for boiling point elevation, we use molality to measure the concentration of the solute because it is temperature independent. Do not forget about the van't Hoff factor, i, in your freezing point calculations.

One way to rationalize the freezing point depression phenomenon without talking about Raoult's law is to consider the freezing process. In order for a liquid to freeze it must achieve a very ordered state that results in the formation of a crystal. If there are impurities in the liquid, i.e. solutes, the liquid is inherently less ordered. Therefore, a solution is more difficult to freeze than the pure solvent so a lower temperature is required to freeze the liquid.

Osmotic Pressure

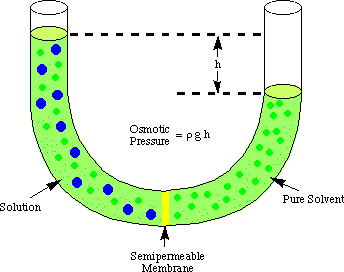
Osmosis refers to the flow of solvent molecules past a semipermeable membrane that stops the flow of solute molecules only. When a solution and the pure solvent used in making that solution are placed on either side of a semipermeable membrane, it is found that more solvent molecules flow out of the pure solvent side of the membrane than solvent flows into the pure solvent from the solution side of the membrane. That flow of solvent from the pure solvent side makes the volume of the solution rise. When the height difference between the two sides becomes large enough, the net flow through the membrane ceases due to the extra pressure exerted by the excess height of the solution chamber. Converting that height of solvent into units of pressure (by using the ) gives a measure of the osmotic pressure exerted on the solution by the pure solvent. P stands for pressure, r is the density of the solution, and h is the height of the solution.

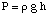
shows a typical setup for measuring the osmotic pressure of a solution.

Figure %: Setup for Measuring the Osmotic Pressure of a Solution

You can understand why more molecules flow from the solvent chamber to the solution chamber in analogy to our discussion of Raoult's law. More solvent molecules are at the membrane interface on the solvent side of the membrane than on the solution side. Therefore, it is more likely that a solvent molecule will pass from the solvent side to the solution side than vice versa. That difference in flow rate causes the solution volume to rise. As the solution rises, by the pressure depth equation, it exerts a larger pressure on the membrane's surface. As that pressure rises, it forces more solvent molecules to flow from the solution side to the solvent side. When the flow from both sides of the membrane are equal, the solution height stops rising and remains at a height reflecting the osmotic pressure of the solution.

The equation relating the osmotic pressure of a solution to its concentration has a form quite similar to the ideal gas law:

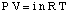
Although the above equation may be more simple to remember, the is more useful. This form of the equation has been derived by realizing that n / V gives the concentration of the solute in units of molarity, M.

P=iMRT